


Next: 4.8 Parameterization of Shortwave
Up: 4. Model Physics
Previous: 4.6 Dry Adiabatic Adjustment
Contents
Cloud amount (or cloud fraction), and the associated optical
properties, are evaluated via a diagnostic method in CAM 3.0. The
basic approach is similar to that employed in the CCM2 and CCM3. The
diagnosis of cloud fraction is a generalization of the scheme
introduced by Slingo [161], with variations described in
Kiehl et al. [91]; Hack et al. [66], and Rasch and Kristjánsson [144]. Cloud fraction depends
on relative humidity, atmospheric stability and convective mass
fluxes. Three types of cloud are diagnosed by the
scheme: low-level marine stratus (
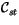 ), convective cloud
(
), convective cloud
(
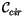 ), and layered cloud (
), and layered cloud (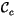 ). Layered clouds form
when the relative humidity exceeds a threshold value which varies
according to pressure. The diagnoses of these cloud types are
described in more detail in the following paragraphs.
). Layered clouds form
when the relative humidity exceeds a threshold value which varies
according to pressure. The diagnoses of these cloud types are
described in more detail in the following paragraphs.
Marine stratocumulus clouds are diagnosed using an empirical
relationship between marine stratocumulus cloud fraction and the
stratification between the surface and 700mb derived by
Klein and Hartmann [95]. The CCM3 parameterization for stratus cloud fraction
over oceans has been replaced with
![$\displaystyle \cfrac _{st} = \min\biggl\lbrace 1., \max\bigl[0., (\theta_{700}-\theta_s)*.057-.5573 \bigr] \biggr\rbrace$](img1663.gif) |
(4.169) |
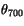 and
and 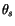 are the potential temperatures at 700 mb
and the surface, respectively. The cloud is assumed to be located in
the model layer below the strongest stability jump between 750 mb and
the surface. If no two layers present a stability in excess of -0.125
K/mb, no cloud is diagnosed. In areas where terrain filtering has
produced non-zero ocean elevations, the sea surface temperature used
for this computation is reduced from the true sea surface elevation to
the model surface elevation according to the lapse rate of the
U.S. Standard Atmosphere (-6.5
are the potential temperatures at 700 mb
and the surface, respectively. The cloud is assumed to be located in
the model layer below the strongest stability jump between 750 mb and
the surface. If no two layers present a stability in excess of -0.125
K/mb, no cloud is diagnosed. In areas where terrain filtering has
produced non-zero ocean elevations, the sea surface temperature used
for this computation is reduced from the true sea surface elevation to
the model surface elevation according to the lapse rate of the
U.S. Standard Atmosphere (-6.5  C/km).
C/km).
Convective cloud fraction in the model is related to updraft mass flux
in the deep and shallow cumulus schemes according to a functional form
suggested by Xu and Krueger [192]:
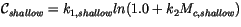 |
(4.170) |
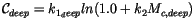 |
(4.171) |
where
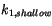 and
and
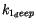 are adjustable parameters given
in Appendix C,
are adjustable parameters given
in Appendix C, 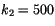 , and
, and 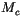 is the
convective mass flux at the given model level.
is the
convective mass flux at the given model level.
The remaining cloud types are diagnosed on the basis of relative
humidity, according to
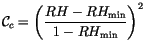 |
(4.172) |
The threshold relative humidity 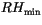 is set according to
pressure
is set according to
pressure 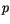 as
as
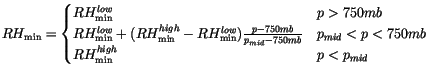 |
(4.173) |
where 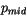 in an adjustable parameter denoting the
minimum pressure for a linear ramp from the low cloud threshold to the
high cloud threshold. At present this ramp is implemented only in one
configuration of the model; other versions have a step function
achieved by setting
in an adjustable parameter denoting the
minimum pressure for a linear ramp from the low cloud threshold to the
high cloud threshold. At present this ramp is implemented only in one
configuration of the model; other versions have a step function
achieved by setting
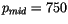 mb.
mb.
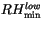 ,
,
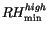 , and
, and 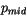 are specified as
in Appendix C. Also, the parameter
are specified as
in Appendix C. Also, the parameter
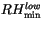 is adjusted over land by
is adjusted over land by 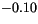 . This distinction
is made to account for the increased sub-grid-scale variability of the water
vapor field due to inhomogeneities in the land surface properties and
subgrid orographic effects.
. This distinction
is made to account for the increased sub-grid-scale variability of the water
vapor field due to inhomogeneities in the land surface properties and
subgrid orographic effects.
The total cloud
 within each volume is then diagnosed as
within each volume is then diagnosed as
This is equivalent to a maximum overlap assumption of cloud types
within each gridbox. The condensate value is assumed uniform within
any and all types of cloud within each grid box.



Next: 4.8 Parameterization of Shortwave
Up: 4. Model Physics
Previous: 4.6 Dry Adiabatic Adjustment
Contents
Jim McCaa
2004-06-22
![]() ), convective cloud
(
), convective cloud
(
![]() ), and layered cloud (
), and layered cloud (![]() ). Layered clouds form
when the relative humidity exceeds a threshold value which varies
according to pressure. The diagnoses of these cloud types are
described in more detail in the following paragraphs.
). Layered clouds form
when the relative humidity exceeds a threshold value which varies
according to pressure. The diagnoses of these cloud types are
described in more detail in the following paragraphs.
![]() is set according to
pressure
is set according to
pressure ![]() as
as
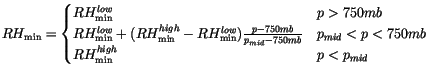
![]() within each volume is then diagnosed as
within each volume is then diagnosed as